Answers to most problems

Last updated

Jan 11, 2021
Save as PDF
- Index
- Tables

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Answers are provided for most problems so you can immediately check your answers to see if you are doing it correctly. This should facilitate learning. Answers are not provided for some problems to simulate real-world conditions and tests, since answers are not known in either case.

Chapter 1

1a. parameter

1b. statistic

2. parameter

4a. $H_0: \mu = 20$ $H_1: \mu > 20$

4c. $H_0: \mu_A = \mu_C$ $H_1: \mu_A \ne \mu_C$

4d. $H_0: p_m = p_A$ $H_1: p_m \ne p_A$

p-value	$\alpha$	Hypothesis $H_0$ or $H_1$	Significant or Not Significant	Error Type I or Type II
0.043	0.05	$H_1$	Significant	Type I
0.32	0.05	$H_0$	Not Significant	Type II
$5.6 \times 10^{-6}$	0.05	$H_1$	Significant	Type I
7.3256	0.01	x	x	x

7a. At the 5% level of significance, the proportion is significantly greater than 0.5 (p = 0.022, n = 350).

7b. At the 1% level of significance, the proportion is not significantly less than 0.25 (p = 0.048, n = 1400).

7d. At the 5% level of significance, the mean is different than 20 ( $5.6 \times 10^{-5}$ , n = 32).

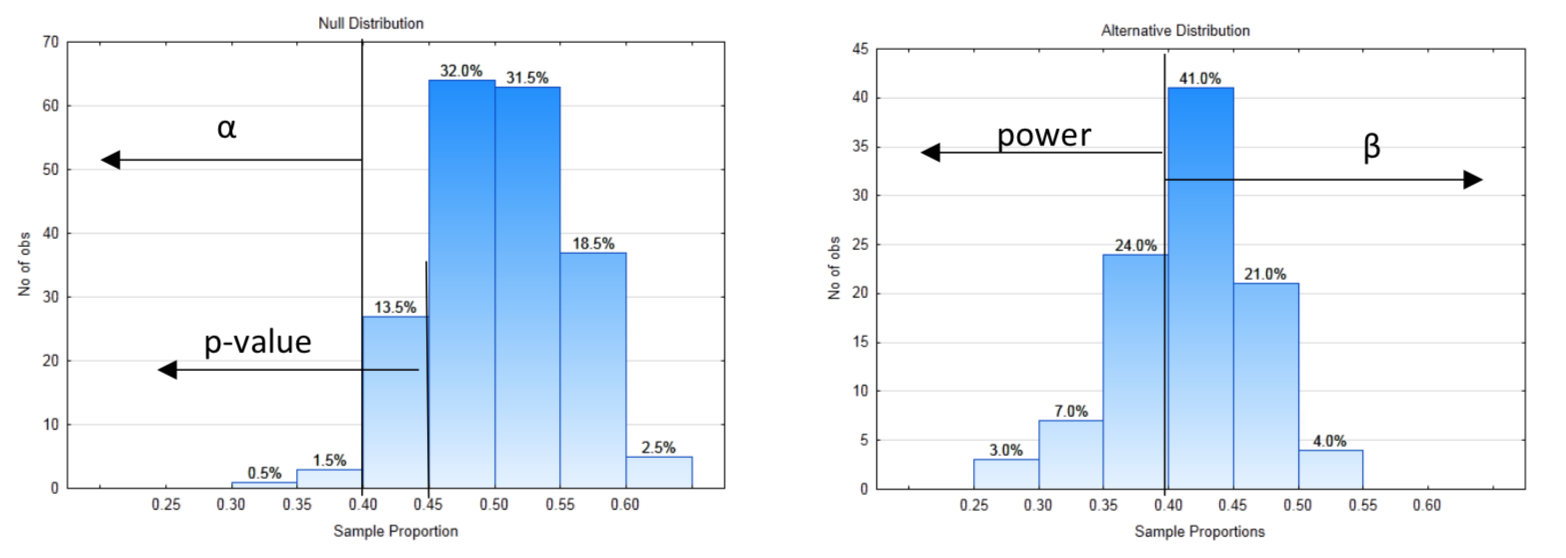
屏幕快照 2019-05-22 下午3.43.27.png

8a. Left

8c. 0.40

8e. 0.66

8h. 0.155

8i. $H_0$

8j. No

8k. type II

8l. At the 2% level of significance, the proportion is not significantly less than 0.5 (p = 0.155, n = 80).

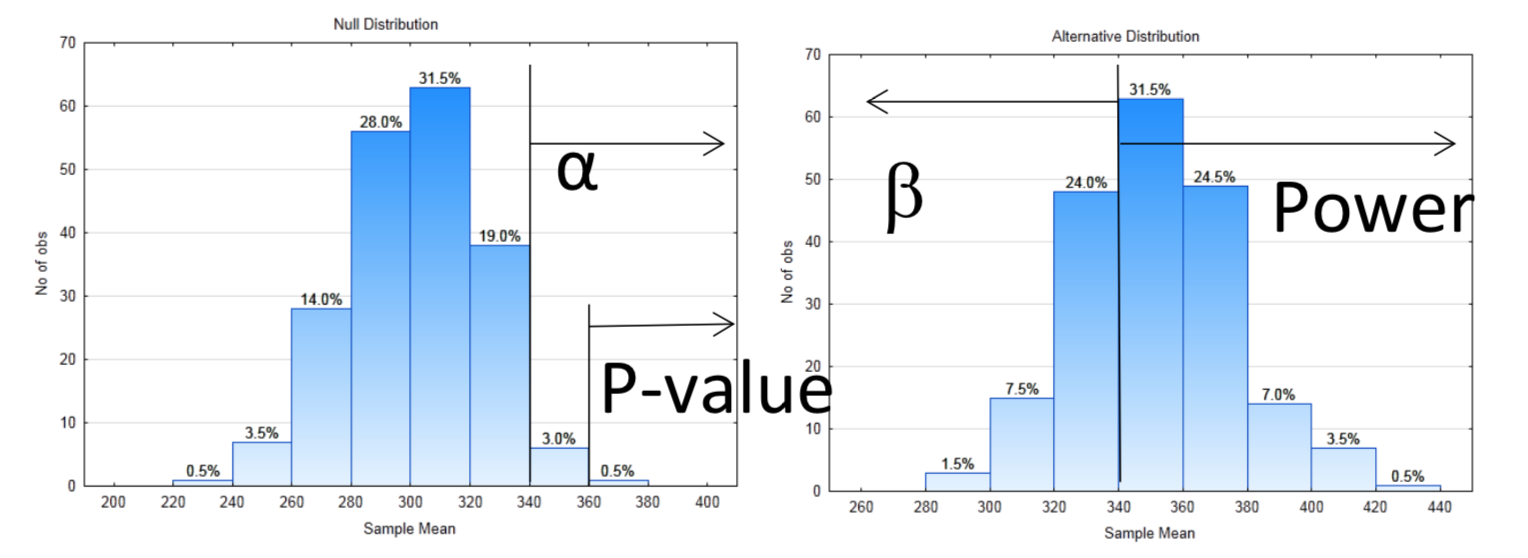
屏幕快照 2019-05-22 下午3.44.47.png

9a. Right

9c. 340

9d. 0.035

9f. 0.67

9h. 0.005

9i. H1

9j. Yes

9l. At the 0.035 level of significance, the mean is significantly greater than 300 (p = 0.005, n = 10).

10a. $H_0: p = 0.5$ $H_1: p > 0.5$

10b. Right

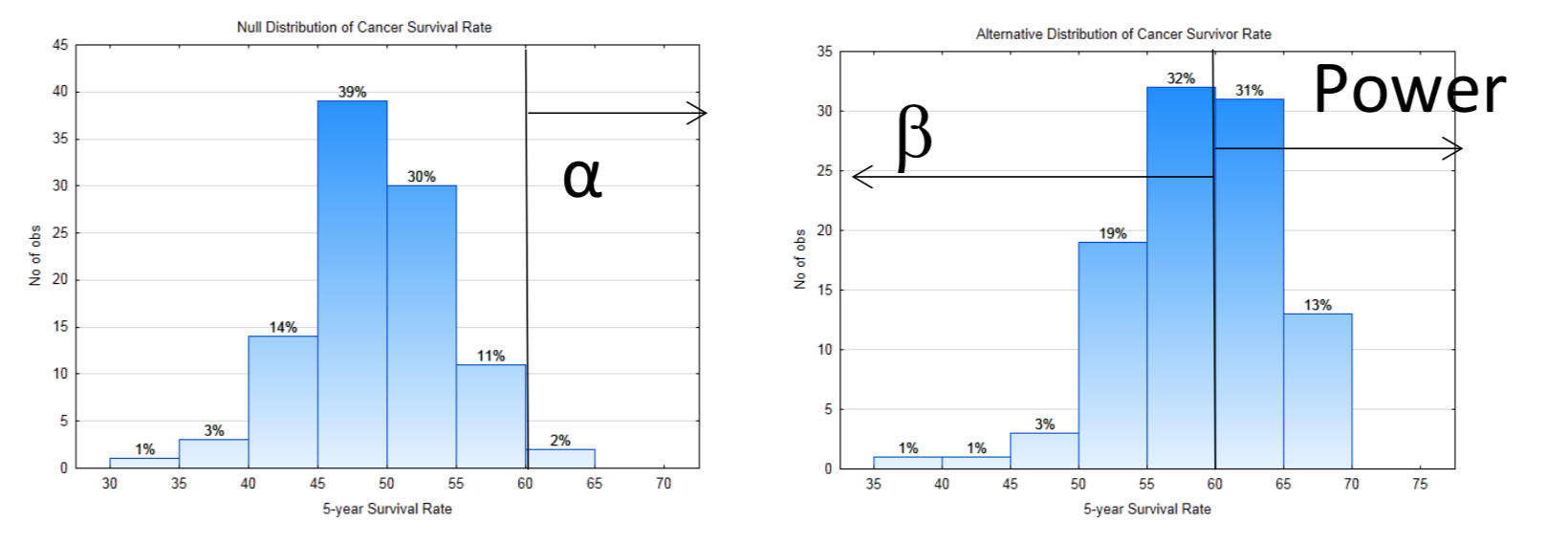
屏幕快照 2019-05-22 下午3.46.28.png

10d. 60

10f. 0.56

10g. 0.44

10h. 0

10i. At the 2% level of significance, the proportion who survive cancer at least 5 years is significantly greater than 0.5 (p = 0, n = 100).

Chapter 2

Research Design Table
Research Question: which route has a faster average time
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	Time it takes for the commute
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential latent variables	Think of at least 2 yourself
Grouping/explanatory Variables 1 (if present) routes	Levels: Route 1 and Route 2

Research Design Table
Research Question: Which is more effective at increasing biodiversity, the hands-off approach or the deliberate approach?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	Number of species
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential latent variables	Think of at least 2 yourself
Grouping/explanatory Variables 1 (if present) approaches	Levels: hands-off deliberate control

4b.

Research Design Table
Research Question: Does static or dynamic stretching result in improvement in flexibility in the largest proportion of people?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	Improvement in sit and reach test
What is the parameter that will be calculated?	Mean Proportion
List potential latent variables	Think of at least 2 yourself
Grouping/explanatory Variables 1 (if present) Stretching method	Levels: static dynamic

8a. 102 N, 40 N, 18 Y, 49 N, 61 N, 60 N, 57 N, 16 N, 90 N, 46 Y,

135 N, 105 Y, 83 N, 102 N, 3 N, 70 Y, 47 N, 42 N, 5 N, 68 N,

Sample Proportion __4/20 = 0.2

8b. West 37 Y,45 N, 21 N, 56 N, 70 Y, 68 N, 65 N, 18 Y, 22 N, 52 Y, 75 Y,

East 93 N, 105 Y, 109 N, 90 N, 114 N, 137 Y, 133 N, 131 N, 104 Y

Sample Proportion _8/20 = 0.4

8c. 2 Y, 9 N, 16 N, 23 N, 30 N, 37 Y, 44 Y, 51 Y, 58 N, 65 N,
72 Y, 79 N, 86 Y, 93 N, 100 N, 107 N, 114 N, 121 N, 128 N, 135 N,

Sample Proportion _6/20 = .30

8d. Which cluster is selected? ___7____ Sample Proportion _9/20=0.45

8m 屏幕快照 2019-05-22 下午3.57.45.png

9a.

Research Design Table
Research Question: Does raising the minimum wage cause unemployment to increase?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	Change in Unemployment rate
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential latent variables	Think of at least 2 yourself
Grouping/explanatory Variables 1 (if present) State minimum wage change	Levels: Increase minimum wage No change in minimum wage

9b. Cluster

9c. 2004, 2012, 2006

9d. Provide your own thoughtful answer.

9e. Provide your own thoughtful answer.

9f. Provide your own thoughtful answer.

9g. At the 5% level of significance, there is not a significant difference in the change in unemployment rate between states that raised their minimum wage and those that didn’t (p = 0.286).

10a.

Research Design Table
Research Question: Will the number of falls increase after bedrails are removed?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	Falls
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential latent variables	Think of at least 2 yourself
Grouping/explanatory Variables 1 (if present) Bedrails	Levels: Present Not present

10b. At the 5% level of significance, there was not a significant increase in the number of falls per 10,000 bed days after the implementation of the new policy (p = 0.18).

10c. There were fewer serious falls, more minor and no-injury falls. A possible reason is that the falls are from a lower height since the patient isn’t crawling over the top of the rails.

10d. Provide your own thoughtful response.

Chapter 3

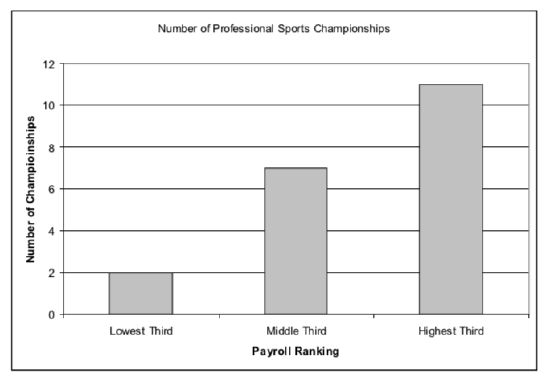
屏幕快照 2019-05-22 下午4.04.11.png

屏幕快照 2019-05-22 下午4.04.42.png

3. mean = 43, Standard deviation = 4.78, variance = 22.89

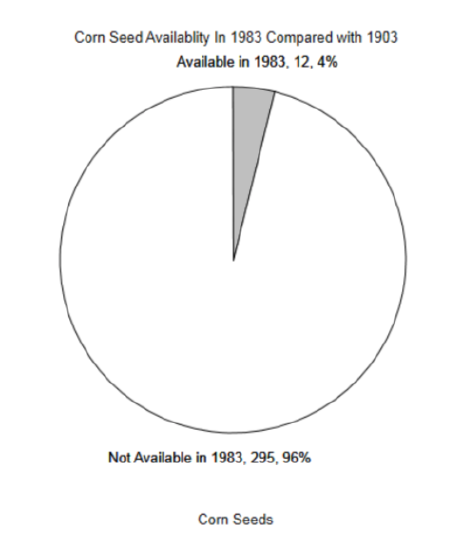
屏幕快照 2019-05-22 下午4.05.48.png

屏幕快照 2019-05-22 下午4.06.10.png

5. $r = \dfrac{\text{cov}(x, y)}{s_x s_y} = \dfrac{2.09}{5.56 \cdot 1.20} = 0.313$

6a.

Research Design Table
Research Question: Is average number of problems answered correctly in one minute was greater for students who passed the class than for those who didn’t pass?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	Number of correctly answered problems
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential latent variables
Grouping/explanatory Variables 1 (if present) Success in course	Levels: Pass Fail
Grouping/explanatory Variables 2 (if present)	Levels:

6b. Calc: 2,9

6c. Cluster

6d. Quantitative discrete

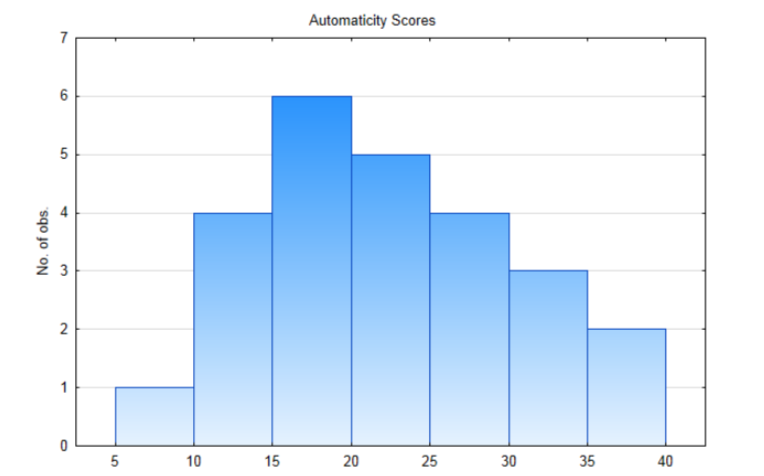
6e.

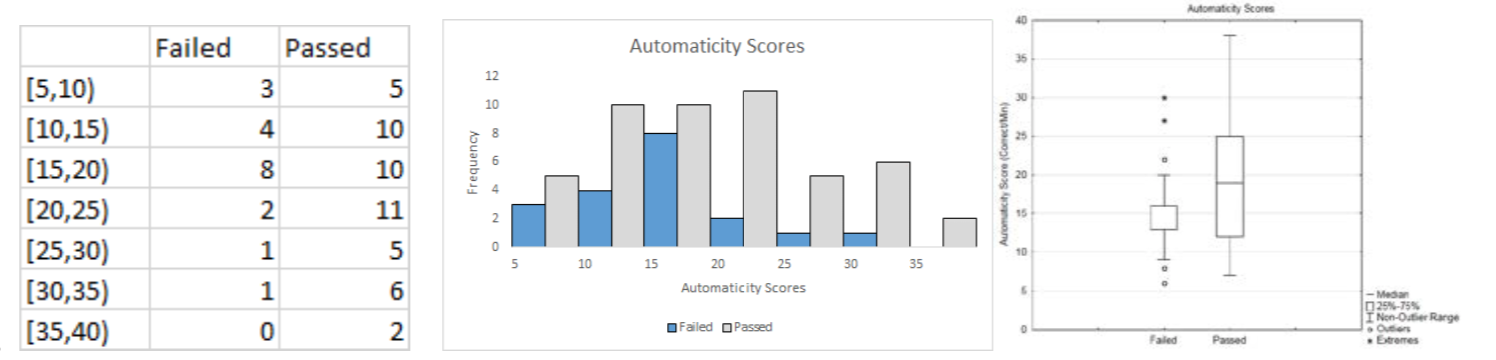
屏幕快照 2019-05-22 下午4.10.36.png

6f. Provide your own thoughtful response.

6g.

	Mean	Variance	Standard Deviation
Failed	15.89	33.88	5.82
Passed

6h. At the 5% level of significance, the average automaticity score of those who pass the class is significantly more than the score of those who fail the class (p = 0.0395, $n_{\text{fail}}$ = 19, $n_{\text{pass}}$ = 49).

6i. Yes

7a.

Research Design Table
Research Question: Is the average heart rate lower with alcohol than with water?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	heart rate
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential latent variables	List you own ideas
Grouping/explanatory Variables 1 (if present) drop	Levels: water (first time) alcohol, water (second time)
Grouping/explanatory Variables 2 (if present)	Levels:

7b.

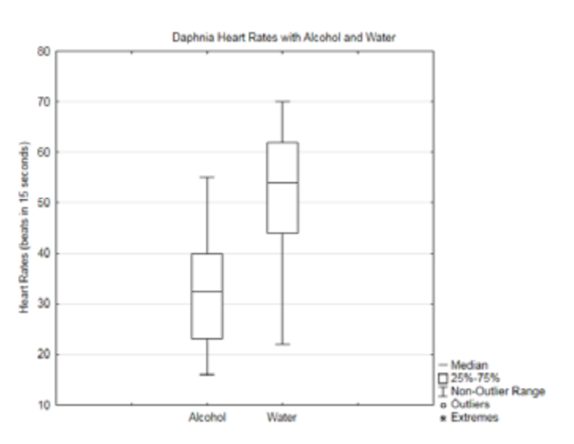
屏幕快照 2019-05-22 下午4.14.19.png

7c.

	Heart Rate after Alcohol	Heart Rate after Water
mean	32.8	52.1
Standard Deviation	10.7	13.0
Median	32.5	54.0

7d. At the 5% level of significance, the average daphnia heart rate with alcohol is significantly less than the average daphnia heart rate with water (p = $1.28 \times 10^{-5}$ , $n_{\text{alcohol}}$ = 18, $n_{\text{water}}$ = 18).

7e. Provide your own thoughtful answer.

Chapter 4

1.
1a. P(S) = 0.70

1b. P(F) = 0.30

1c. P(FSSSF) = P(F)P(S) P(S) P(S) P(F) = (0.3) (0.7) (0.7) (0.7) (0.3) = 0.03087

1d. 10

1e. 0.3087

1f.

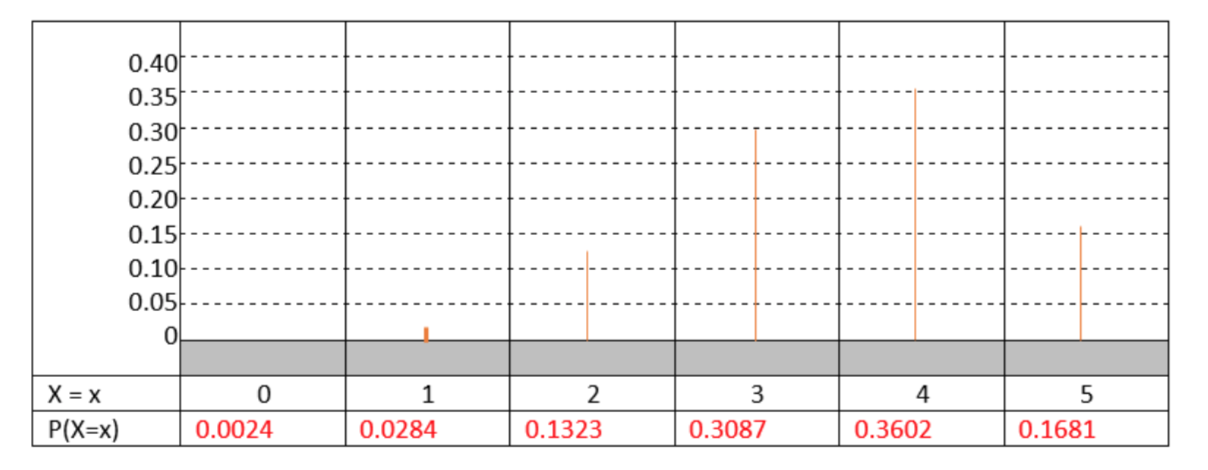
屏幕快照 2019-05-22 下午4.17.49.png

1g. $\mu = np = 5(0.7) = 3.5$ $\sigma = \sqrt{npq} = \sqrt{5 (0.7) (0.3)} = 1.025$

1h. $P(X \le 3)$ = binomcdf(n, p, x) = binomcdf (5, 0.70, 3) = 0.4718. The null is supported. The data are not significant.

2a. P(S) = 0.40

2b. P(F) = 0.60

2c. 0.0036864

2d. 21

2e. 0.0774

2f.

$X = x$	0	1	2	3	4	5	6	7
$P(X = x)$	0.0280	0.1306	0.2613	0.2903	0.1935	0.0774	0.0172	0.0016

2g. 2.8, 1.296

2h. P-value = 0.0963 alternative

3j. P-value = 0.047

At the 5% level of significance, the proportion of residents opposed to the terminals is significantly greater than 0.5 (p = 0.047, n = 300)

3k. z = 1.73 p-value 0.0418

At the 5% level of significance, the proportion of residents opposed to the terminals is significantly greater than 0.5 (z = 1.73, p = 0.0418, n = 300).

3l. 0.55

3m. 0.02887

3n. z = 1.73 p-value 0.0418

At the 5% level of significance, the proportion of residents opposed to the terminals is significantly greater than 0.5 (z = 1.73, p = 0.0418, n = 300).

4a. $H_0: \mu = 43,362$ and $H_1: \mu < 43,362$

4b. $\mu = 43,362$

4c. $\sigma_{\bar{x}} = \dfrac{\sigma}{\sqrt{n}} = \dfrac{7900}{\sqrt{10}} = 2498$

4d.

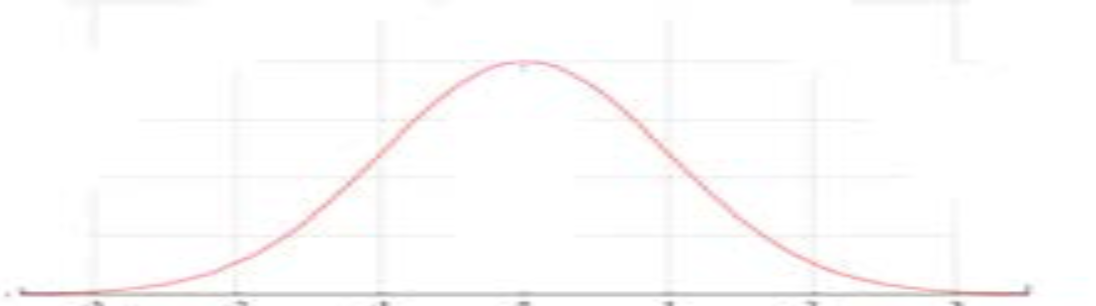
屏幕快照 2019-05-22 下午4.25.12.png

X axis: 35868 38366 40864 43362 45860 48358 50856

4e. 18,225 (use stat-edit and then stat-calc-1-var stats)

4f. z = -10.06 p-value < 0.0002

6b. $H_0: \mu = 54.1$ and $H_1: \mu > 54.1$

6d. $bar{x} = 46.73$ , $s = 16.377$

6e. $\mu = 54.1$ $\sigma_{\bar{x}} = 2.939$

6f.

屏幕快照 2019-05-22 下午4.25.12.png

45.4 48.3 51.2 54.1 57 59.9 62.8

6g. z = -2.51 p-value 0.9940

At the 5% level of significance, the average walk score of small cities is not significantly greater than big cities (z = -2.51, p = 0.994, n = 30)

7e. p-value = 0.3865 0.0148

7g p-value = 0.0129

7h z = 2.229, p= 0.0129

8c.

	Impact	Control
Mean	455
Standard Deviation	614.8
Median	150

Chapter 5

1a. $H_0: \mu_T = \mu_D$ $H_1: \mu_T \ne \mu_D$ Test: 2 independent samples t test

1b. $H_0: p = 0.5$ $H_1: p > 0.5$ Test: 1 proportion z test

1c. $H_0: p_{STEM} = p_{SS}$ $H_1: p_{STEM} \ne p_{SS}$ Test: 2 proportion Z test

1e. $H_0: \mu = 7$ $H_1: \mu > 7$ Test: 1 sample t test

1g. $H_0: \mu = 0$ $H_1: \mu < 0$ Test: 1 sample t test

2a. $H_0: \mu = 15$ , $H_1: \mu < 15$

2b. Test the bypothesis:

$t = \dfrac{\bar{x} - \mu}{\dfrac{s}{\sqrt{n}}}$ $t = \dfrac{14.28 - 15}{\dfrac{4.6}{\sqrt{30}}}$ t = -0.857 p > 0.1 or p = 0.1991

Formula Substitution Test Statistic p-value

2c. Fill in the blanks for the concluding sentence. At the _5%__ level of significance, the mean money spent per day _is not_ significantly less than $15 (t = ____________, p ___________, df = 29).

3a. Write the hypotheses: $H_0: p = 0.5$ , $H_1: p > 0.5$ , Sample proportion $(\hat{p}$ ) = $\dfrac{118}{179}$ = 0.659

3b. Test the hypothesis:

$z = \dfrac{\hat{p} - p}{\sqrt{\dfrac{p(1 - p}{n}}}$ $z = \dfrac{0.659 - 0.5}{\sqrt{\dfrac{0.5(1 - 0.5}{179}}}$ p < 0.0002 or 1.02 $\times 10^{-5}$

Formula Substitution Test Statistic p-value

Write the concluding sentence: At the 5% level of significance, the average price on Tuesday is not significantly less than other days (t = -0.479, p > 0.25, n = 7).

5a. Write the hypotheses: $H_0:$ _ $\mu_{40} = \mu_{60}$ ___, $H_1:$ __ $\mu_{40} < \mu_{60}$ __,

5c. Write the concluding sentence: At the 10% level of significance, the mean guess at Morocco’s population by people with low phone digits is significantly less than the mean guess of those with high phone digits ( $t$ = -1.835, $p$ < 0.05, $n_{40}$ = 15, $n_{60}$ = 15).

6c. Test the hypothesis: Test Statistic = -12.41, p-value = p =1

7e. Write a concluding sentence. At the 5% level of significance, the proportion of $12^{\text{th}}$ grade students using drugs in 2012 is not significantly greater than in 2002 ( $z$ = 0.819, $p$ = 0.2061, $n_{2012}$ = 630, $n_{2002}$ = 2184).

8a.

Research Design Table
Research Question: Is there a significant difference in the mean times of the men and women who finish the triathlon course?
Type of Research	Observational Study Observational Experiment Manipulative Experiment
What is the response variable?	heart rate
What is the parameter that will be calculated?	Mean Proportion Correlation
List potential confounding variables
Grouping/explanatory Variables 1 (if present) Gender	Levels: Men, Women

8b. Write the hypotheses. $H_0: \mu_{\text{men}} = \mu_{\text{women}}$ , $H_1: \mu_{\text{men}} \ne \mu_{\text{women}}$

9a. Conclusion: At the 5% level of significance, there is not a significant difference in the proportion of days that African Americans and LGB individuals record at least one stigma-related stressor ( $z$ = 0.062, $p = 0.950$ , $n_{AA}$ = 190, $n_{LGB}$ = 310)

9b. Conclusion: At the 5% level of significance, the mean psychological distress score for those using rumination is significantly different than for those using distraction ( $t$ = 2.189, $p$ = 0.033, $n_R$ = 26, $n_D$ = 26)

Chapter 6

1. 0.778

Margin of Error 0.060 Confidence Interval (0.718,0.838) Calculator confidence interval (0.71853,0.83823)

2. Girls: 0.743, Boys 0.778

Margin of Error 0.132, Confidence Interval (9-0.167,0.097)

3. (Note: There are 112 degrees of freedom. This df does not appear in your tables. It falls between 60 df and 120 df. To make sure that the interval is sufficiently large, the critical t value for 60 df will be used. The actual value, as found using the Excel function T.INV.2T(0.1,112) is 1.6586).

Margin of error 14.6 (73.4,102.6)

Calculator confidence interval (73.49,102.51)

5. Point estimate 156

Margin of error 92.9 Confidence Interval (63.1,248.9)

Calculator confidence interval (63.087,248.91)

6. Point Estimate 5.2 degrees

Margin of error 3.2 confidence interval (2,8.4)

Calculator confidence interval (2.0338,8.3662)

8. Point estimate 0.116

Margin of Error 0.017, Confidence interval (0.099,0.133)

Margin of Error	1%	5%	10%	20%
Sample Size	9604

10.

Degree of Confidence	99%	95%	90%	80%
Sample Size	1844

11a. Stratified

11b. 256 , 379 ,

11d. Mean = 59.5, SD = 23.78

11e. (48.4, 70.6)

11f. Lowest: 290.4

Chapter 7

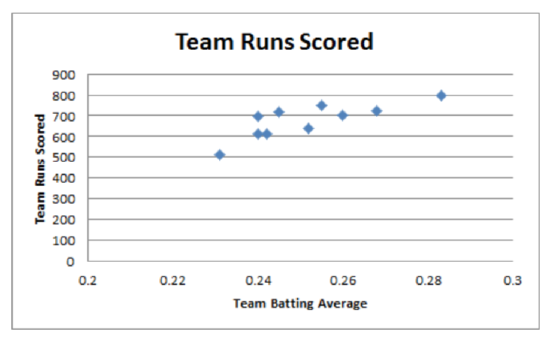
1b Mean batting average 0.2516 Standard deviation for batting average 0.0155

Mean runs scored 676.1 Standard deviation for runs scored 82.20

1c. At the 5% level of significance, there is a significant correlation between batting average and runs scored ( $t$ = 3.84, $p$ < 0.01, $n$ = 10).

1d. Regression equation: y = -397.98 + 4269x

1e. $r^2$ = 0.6479. It means 64.8% of the total variation from the mean for team runs scored is attributed to the variation in the batting average.

1f. 669.285

1g.

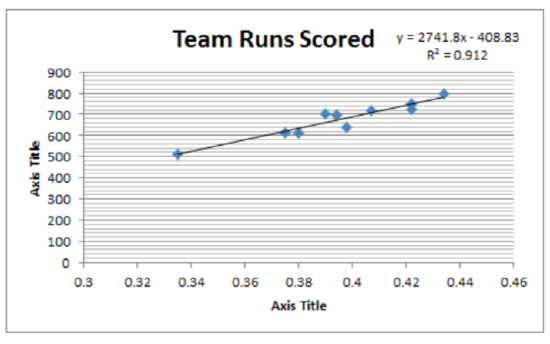
屏幕快照 2019-05-23 下午1.16.39.png

Correlation: 0.955

Hypothesis test concluding sentence: At the 5% level of significance, there is a significant correlation between slugging percentage and runs scored ( $t$ = 9.10, $p$ = 1.699E-5, $n$ = 10).

Regression equation: $y = -408.83 + 2741.80x$

Coefficient of determination ( $r^2$ ): 0.912

Predict the number of runs scored for a team with a slugging percentage of 0.400. 687.89

2a.

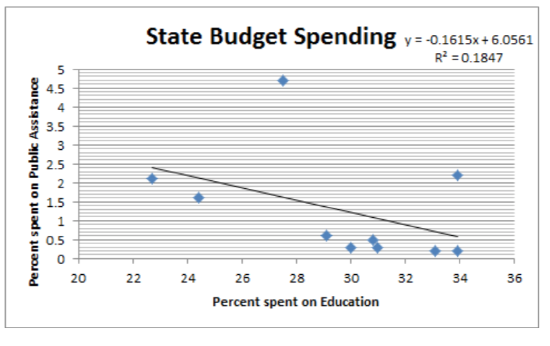
屏幕快照 2019-05-23 下午1.18.34.png

2b.

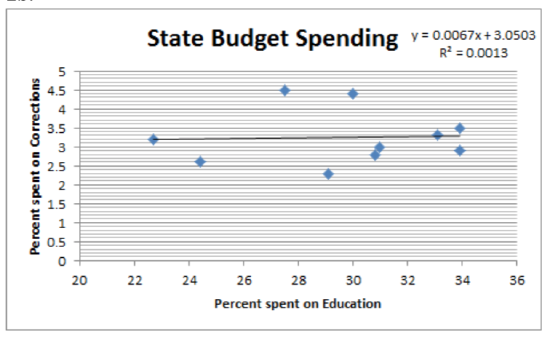
屏幕快照 2019-05-23 下午1.18.56.png

$R$ = 0.0359, $r^2$ = 0.0013, $y = 3.05 + 0.0067x$

At the 5% level of significance, there is not a significant correlation between spending on education and spending on public assistance ( $t$ = -1.35, $p$ = 0.215, $n$ = 10).

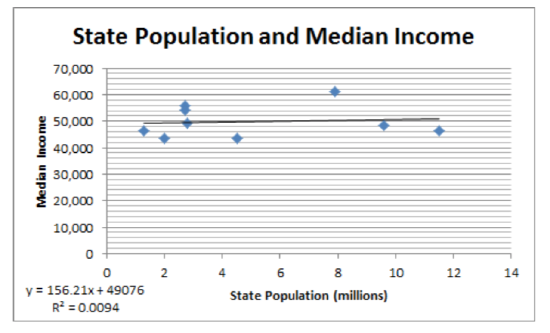
屏幕快照 2019-05-23 下午1.20.04.png

$Y = 49075.6 +156.2x$ $r = 0.097$ , $r^2 = 0.0094$ , $t = 0.258$ , $p = 0.8038$ .

4a.

屏幕快照 2019-05-23 下午1.21.17.png

$Y=-36.38+0.0023x$ $r = 0.929$ , $r^2 = 0.863$ , $t = 6.64$ , $p = 2.92 \times 10^{-4}$

4b. Provide your own thoughtful response.

4c. 101.62

5a. Age

5b. NPA

5c. 2, 12, 22, 32, 42, 52, 62, 72, 82, 92, 102

5e. r = 0.814

5f. At the 5% level of significance, there is a significant correlation between age and NPA ( $t$ = 4.2, $p$ = 0.002, $n$ = 11).

Chapter 8

1a. Test for Homogeneity

1b. Goodness of Fit
1c. Test for Independence

1d. Goodness of Fit

$X = x$	0	1	2	3
$P(X = x)$	0.5787	0.34722	0.06944	0.00463

Goodness of Fit

$\chi^2 = 3.43$

At the 5% level of significance, there is not a significant difference between the observed and expected distributions ( $\chi^2 = 3.43$ , $p > 0.1$ , $n = 158$ ) . Calculator p-value is 0.33.

3. Test for Independence
$\chi^2$ = 13.27
At the 0.1 level of significance there is a correlation between shots and goals ( $\chi^2 = 13.27$ , $p < 0.005$ , $n = 49$ ) (calculator p-value = $2.696 \times 10^{-4}$ ).

4. Test for Homogeneity

At the 0.1 level of significance, the distributions for improvement from drug and non-drug treatments are homogeneous ( $\chi^2 = 0.8222$ , $p > 0.1$ , $n = 80$ ) (Calculator: $p = 0.6629$ , df=2)
5. $\chi^2 = 9.426$

6a. stratified

6b. 2042, 584, _____

6c. Goodness of Fit

6d.

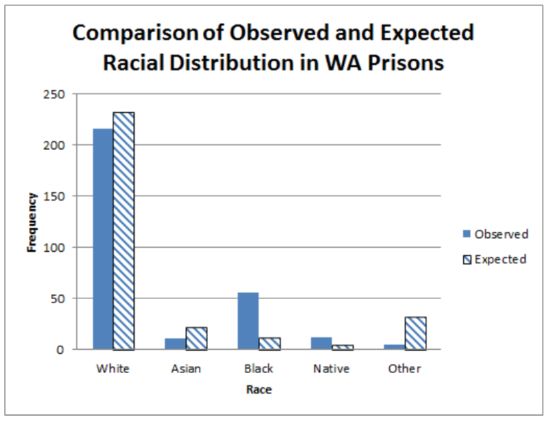
屏幕快照 2019-05-23 下午1.28.36.png

6f. At the 5% level of significance, the racial distribution of WA prisons is significantly different than what would be expected ( $\chi^2 = 229.96$ , $p < 0.005$ , $n = 300$ ) (Calculator: $p = 1.3 \times 10^{-48}$ , df = 4).

Search

Text Color

Text Size

Margin Size

Font Type

Support Center

How can we help?