4.6E: Exercises for Section 4.5

Last updated
Save as PDF

Page ID: 25965

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

1) If $c$ is a critical point of $f(x)$, when is there no local maximum or minimum at $c$? Explain.

2) For the function $y=x^3$, is $x=0$ both an inflection point and a local maximum/minimum?

Answer: It is not a local maximum/minimum because $f'$ does not change sign

3) For the function $y=x^3$, is $x=0$ an inflection point?

4) Is it possible for a point $c$ to be both an inflection point and a local extremum of a twice differentiable function?

Answer: No

5) Why do you need continuity for the first derivative test? Come up with an example.

6) Explain whether a concave-down function has to cross $y=0$ for some value of $x$.

Answer: False; for example, $y=\sqrt{x}$.

7) Explain whether a polynomial of degree $2$ can have an inflection point.

In exercises 8 - 12, analyze the graphs of $f'$, then list all intervals where $f$ is increasing or decreasing.

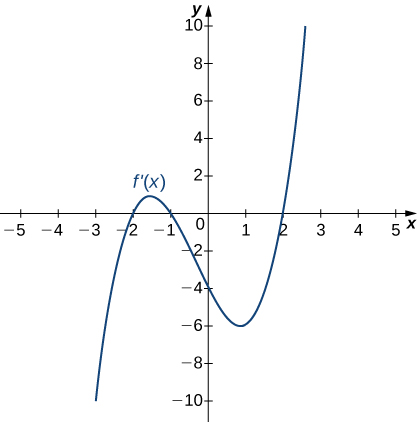
$The function f’(x) is graphed. The function starts negative and crosses the x axis at (−2, 0). Then it continues increasing a little before decreasing and crossing the x axis at (−1, 0). It achieves a local minimum at (1, −6) before increasing and crossing the x axis at (2, 0).$

Answer: Increasing for $−2<x<−1$ and $x>2$;
Decreasing for $x<−2$ and $−1<x<2$

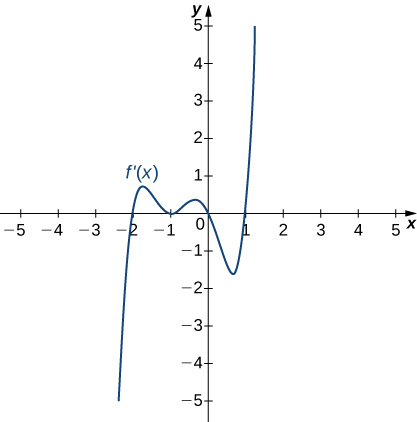
$The function f’(x) is graphed. The function starts negative and crosses the x axis at (−2, 0). Then it continues increasing a little before decreasing and touching the x axis at (−1, 0). It then increases a little before decreasing and crossing the x axis at the origin. The function then decreases to a local minimum before increasing, crossing the x-axis at (1, 0), and continuing to increase.$

10)

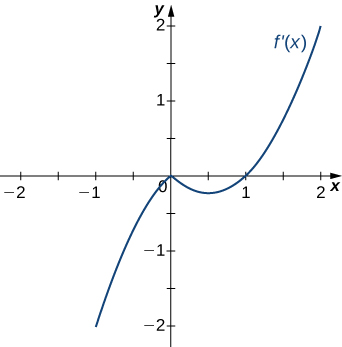
$The function f’(x) is graphed. The function starts negative and touches the x axis at the origin. Then it decreases a little before increasing to cross the x axis at (1, 0) and continuing to increase.$

Answer: Decreasing for $x<1$,
Increasing for $x>1$

11)

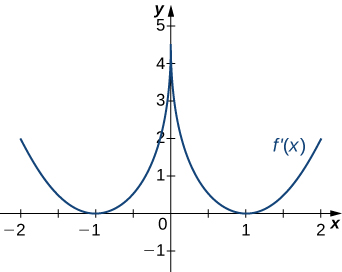
$The function f’(x) is graphed. The function starts positive and decreases to touch the x axis at (−1, 0). Then it increases to (0, 4.5) before decreasing to touch the x axis at (1, 0). Then the function increases.$

12)

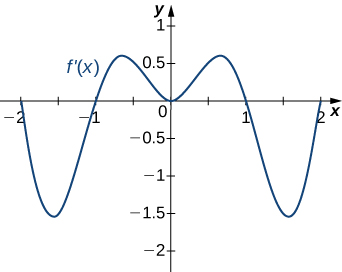
$The function f’(x) is graphed. The function starts at (−2, 0), decreases to (−1.5, −1.5), increases to (−1, 0), and continues increasing before decreasing to the origin. Then the other side is symmetric: that is, the function increases and then decreases to pass through (1, 0). It continues decreasing to (1.5, −1.5), and then increase to (2, 0).$

Answer: Decreasing for $−2<x<−1$ and $1<x<2$;
Increasing for $−1<x<1$ and $x<−2$ and $x>2$

In exercises 13 - 17, analyze the graphs of $f'$, then list all intervals where

a. $f$ is increasing and decreasing and

b. the minima and maxima are located.

13)

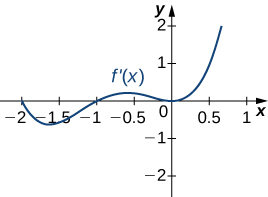
$The function f’(x) is graphed. The function starts at (−2, 0), decreases for a little and then increases to (−1, 0), continues increasing before decreasing to the origin, at which point it increases.$

14)

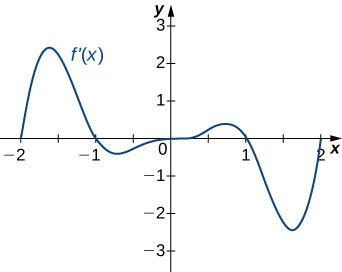
$The function f’(x) is graphed. The function starts at (−2, 0), increases and then decreases to (−1, 0), decreases and then increases to an inflection point at the origin. Then the function increases and decreases to cross (1, 0). It continues decreasing and then increases to (2, 0).$

Answer: a. Increasing over $−2<x<−1,\;0<x<1,x>2$, Decreasing over $x<−2, \;−1<x<0, \;1<x<2;$
b. Maxima at $x=−1$ and $x=1$, Minima at $x=−2$ and $x=0$ and $x=2$

15)

$The function f’(x) is graphed from x = −2 to x = 2. It starts near zero at x = −2, but then increases rapidly and remains positive for the entire length of the graph.$

16)

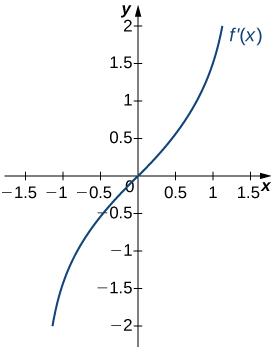
$The function f’(x) is graphed. The function starts negative and crosses the x axis at the origin, which is an inflection point. Then it continues increasing.$

Answer: a. Increasing over $x>0$, Decreasing over $x<0;$
b. Minimum at $x=0$

17)

$The function f’(x) is graphed. The function starts negative and crosses the x axis at (−1, 0). Then it continues increasing a little before decreasing and touching the x axis at the origin. It increases again and then decreases to (1, 0). Then it increases.$

In exercises 18 - 22, analyze the graphs of $f'$, then list all inflection points and intervals $f$ that are concave up and concave down.

18)

$The function f’(x) is graphed. The function is linear and starts negative. It crosses the x axis at the origin.$

Answer: Concave up for all $x$,
No inflection points

19)

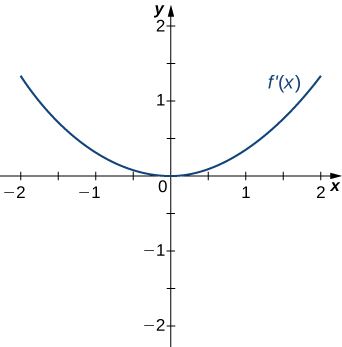
$The function f’(x) is graphed. It is an upward-facing parabola with 0 as its local minimum.$

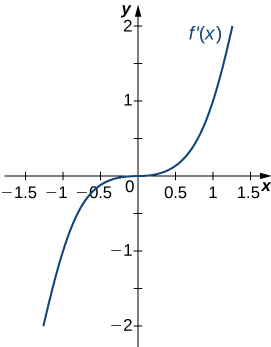
20)

$The function f’(x) is graphed. The function resembles the graph of x3: that is, it starts negative and crosses the x axis at the origin. Then it continues increasing.$

Answer: Concave up for all $x$,
No inflection points

21)

$The function f’(x) is graphed. The function starts negative and crosses the x axis at (−0.5, 0). Then it continues increasing to (0, 1.5) before decreasing and touching the x axis at (1, 0). It then increases.$

22)

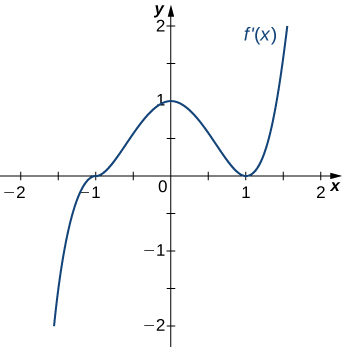
$The function f’(x) is graphed. The function starts negative and crosses the x axis at (−1, 0). Then it continues increasing to a local maximum at (0, 1), at which point it decreases and touches the x axis at (1, 0). It then increases.$

Answer: Concave up for $x<0$ and $x>1$,
Concave down for $0<x<1$,
Inflection points at $x=0$ and $x=1$

For exercises 23 - 27, draw a graph that satisfies the given specifications for the domain $x=[−3,3]$. The function does not have to be continuous or differentiable.

23) $f(x)>0,\;f'(x)>0$ over $x>1,\;−3<x<0,\;f'(x)=0$ over $0<x<1$

24) $f'(x)>0$ over $x>2,\;−3<x<−1,\;f'(x)<0$ over $−1<x<2,\;f''(x)<0$ for all $x$

Answer: Answers will vary

25) $f''(x)<0$ over $−1<x<1,\;f''(x)>0,\;−3<x<−1,\;1<x<3,$ local maximum at $x=0,$ local minima at $x=±2$

26) There is a local maximum at $x=2,$ local minimum at $x=1,$ and the graph is neither concave up nor concave down.

Answer: Answers will vary

27) There are local maxima at $x=±1,$ the function is concave up for all $x$, and the function remains positive for all $x.$

For the following exercises, determine

a. intervals where $f$ is increasing or decreasing and

b. local minima and maxima of $f$.

28) $f(x)=\sin x+\sin^3x$ over $−π<x<π$

Answer

a. Increasing over $−\frac{π}{2}<x<\frac{π}{2},$ decreasing over $x<−\frac{π}{2},\; x>\frac{π}{2}$

b. Local maximum at $x=\frac{π}{2}$; local minimum at $x=−\frac{π}{2}$

29) $f(x)=x^2+\cos x$

For exercise 30, determine

a. intervals where $f$ is concave up or concave down, and

b. the inflection points of $f$.

30) $f(x)=x^3−4x^2+x+2$

Answer

a. Concave up for $x>\frac{4}{3},$ concave down for $x<\frac{4}{3}$

b. Inflection point at $x=\frac{4}{3}$

For exercises 31 - 37, determine

a. intervals where $f$ is increasing or decreasing,

b. local minima and maxima of $f$,

c. intervals where $f$ is concave up and concave down, and

d. the inflection points of $f$.

31) $f(x)=x^2−6x$

32) $f(x)=x^3−6x^2$

Answer: a. Increasing over $x<0$ and $x>4,$ decreasing over $0<x<4$
b. Maximum at $x=0$, minimum at $x=4$
c. Concave up for $x>2$, concave down for $x<2$
d. Inflection point at $x=2$

33) $f(x)=x^4−6x^3$

34) $f(x)=x^{11}−6x^{10}$

Answer: a. Increasing over $x<0$ and $x>\frac{60}{11}$, decreasing over $0<x<\frac{60}{11}$
b. Maximum at $x=0$, minimum at $x=\frac{60}{11}$
c. Concave down for $x<\frac{54}{11}$, concave up for $x>\frac{54}{11}$
d. Inflection point at $x=\frac{54}{11}$

35) $f(x)=x+x^2−x^3$

36) $f(x)=x^2+x+1$

Answer: a. Increasing over $x>−\frac{1}{2}$, decreasing over $x<−\frac{1}{2}$
b. Minimum at $x=−\frac{1}{2}$
c. Concave up for all $x$
d. No inflection points

37) $f(x)=x^3+x^4$

For exercises 38 - 47, determine

a. intervals where $f$ is increasing or decreasing,

b. local minima and maxima of $f$,

c. intervals where $f$ is concave up and concave down, and

d. the inflection points of $f$. Sketch the curve, then use a calculator to compare your answer. If you cannot determine the exact answer analytically, use a calculator.

38) [T] $f(x)=\sin(πx)−\cos(πx)$ over $x=[−1,1]$

Answer: a. Increases over $−\frac{1}{4}<x<\frac{3}{4},$ decreases over $x>\frac{3}{4}$ and $x<−\frac{1}{4}$
b. Minimum at $x=−\frac{1}{4}$, maximum at $x=\frac{3}{4}$
c. Concave up for $−\frac{3}{4}<x<\frac{1}{4}$, concave down for $x<−\frac{3}{4}$ and $x>\frac{1}{4}$
d. Inflection points at $x=−\frac{3}{4},\;x=\frac{1}{4}$

39) [T] $f(x)=x+\sin(2x)$ over $x=[−\frac{π}{2},\frac{π}{2}]$

40) [T] $f(x)=\sin x+\tan x$ over $(−\frac{π}{2},\frac{π}{2})$

Answer: a. Increasing for all $x$
b. No local minimum or maximum
c. Concave up for $x>0$, concave down for $x<0$
d. Inflection point at $x=0$

41) [T] $f(x)=(x−2)^2(x−4)^2$

42) [T] $f(x)=\dfrac{1}{1−x},\quad x≠1$

Answer: a. Increasing for all $x$ where defined
b. No local minima or maxima
c. Concave up for $x<1$; concave down for $x>1$
d. No inflection points in domain

43) [T] $f(x)=\dfrac{\sin x}{x}$ over $x=[-2π,0)∪(0,2π]$

44) $f(x)=\sin(x)e^x$ over $x=[−π,π]$

Answer: a. Increasing over $−\frac{π}{4}<x<\frac{3π}{4}$, decreasing over $x>\frac{3π}{4},\;x<−\frac{π}{4}$
b. Minimum at $x=−\frac{π}{4}$, maximum at $x=\frac{3π}{4}$
c. Concave up for $−\frac{π}{2}<x<\frac{π}{2}$, concave down for $x<−\frac{π}{2},\;x>\frac{π}{2}$
d. Inflection points at $x=±\frac{π}{2}$

45) $f(x)=\ln x\sqrt{x},\quad x>0$

46) $f(x)=\frac{1}{4}\sqrt{x}+\frac{1}{x},\quad x>0$

Answer: a. Increasing over $x>4,$ decreasing over $0<x<4$
b. Minimum at $x=4$
c. Concave up for $0<x<8\sqrt[3]{2}$, concave down for $x>8\sqrt[3]{2}$
d. Inflection point at $x=8\sqrt[3]{2}$

47) $f(x)=\dfrac{e^x}{x},\quad x≠0$

In exercises 48 - 52, interpret the sentences in terms of $f$, $f'$, and $f''$.

48) The population is growing more slowly. Here $f$ is the population.

Answer: $f>0,\;f'>0,\;f''<0$

49) A bike accelerates faster, but a car goes faster. Here $f=$ Bike’s position minus Car’s position.

50) The airplane lands smoothly. Here $f$ is the plane’s altitude.

Answer: $f>0,\;f'<0,\;f''>0$

51) Stock prices are at their peak. Here $f$is the stock price.

52) The economy is picking up speed. Here $f$ is a measure of the economy, such as GDP.

Answer: $f>0,\;f'>0,\;f''>0$

For exercises 53 - 57, consider a third-degree polynomial $f(x)$, which has the properties $f'(1)=0$ and $f'(3)=0$.

Determine whether the following statements are true or false. Justify your answer.

53) $f(x)=0$ for some $1≤x≤3$.

54) $f''(x)=0$ for some $1≤x≤3$.

Answer: True, by the Mean Value Theorem

55) There is no absolute maximum at $x=3$.

56) If $f(x)$ has three roots, then it has $1$ inflection point.

Answer: True, examine derivative

57) If $f(x)$ has one inflection point, then it has three real roots.

Search

Text Color

Text Size

Margin Size

Font Type