10.7: Leases
- Page ID
- 31038
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Average vehicle costs in North America have risen from $10,668 in 1982 to $25,683 in 2009,1 a whopping 141% increase over 27 years. With vehicle purchase prices running well ahead of inflation, leasing now represents an attractive option for many Canadians with many added conveniences. On the upside, lease payments are substantially lower than purchase payments. As a bonus you are able to return your vehicle after a certain number of years and upgrade to the most recent models available. And while you are in possession of the vehicle, it usually remains under the manufacturer warranty, which minimizes your maintenance and repair expenses. On the downside, you never actually own the vehicle and always make car payments, while various research has also calculated that leasing is actually much more expensive than vehicle ownership in the long run.
Businesses take advantage of leasing not only for vehicles, but for real estate and office space along with production equipment and office equipment. Businesses constantly need to change to avoid obsolescence, particularly in their fleet of computers. As a business grows, it must expand or even change its office space. Leasing allows organizations to do all of this. Leasing also offers significant tax benefits to most companies as well.
This section explains the basic principles of a lease and then illustrates leasing calculations such as purchase prices, residual values, lease payments, and leasing interest rates.
How Leases Work
A lease is a contract by which the owner of an asset gives another party an exclusive right to possess and use the asset under specified conditions for a specific period of time in return for agreed-upon payments. In other words, you are able to borrow something for a period of time and make payments on it. At the end of the lease, the asset is usually returned to the owner, or it can be purchased outright for a depreciated price. The owner of the asset is known as the lessor, while the borrowing party is known as the lessee.
A typical leasing timeline is illustrated below. Important lease components include the present value, annuity timing, term, interest rate, and future value.
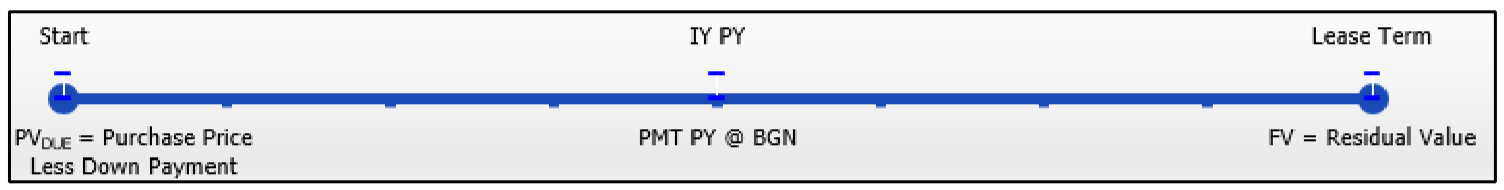
- Present Value of the Lease. The purchase price of an asset is known by many names, such as its selling price, list price, or even the manufacturer's suggested retail price (MSRP). A down payment, which is a portion of the purchase price required up front, may or may not be required depending on the asset being leased. If it is required, it is deducted from the purchase price to determine the amount of money being financed.
- Annuity Timing. All leases take the form of annuities due since you must first pay for the item before you are allowed to borrow it. Car dealerships will not let you drive that new car home until you demonstrate the financial capability to pay for it!
- Lease Term. A lease term is no different from an annuity term. It is the specified period of time for which the lessor leases the asset.
- Lease Interest Rate. The nominal interest rate and compounding frequency are determined by the owner of the asset. Most vehicle leases default to rates that are compounded monthly; however, advertisements typically present effective rates.
- Future Value of the Lease. The future value is more commonly known as the residual value, which is the projected value of an asset at the end of its lease term. A residual value does not necessarily occur in every lease calculation, as some assets, like computer equipment, may only have an insubstantial value upon lease expiration. Vehicle leases always have a residual value. Thus, the lessee has a choice to give the car dealership either the keys to the car or a cheque in the amount of the residual value, thereby purchasing the car.
In accounting, under certain circumstances, when an officer of the company signs a lease for the acquisition of a capital good (i.e., a lease of an asset, not a rental of property like a building), this creates a debt. Most leases are closed and cannot be canceled without severe financial penalties; therefore, a liability is recorded on the balance sheet. The amount recorded is the present value of the remaining lease payments, and it is calculated using a discount rate equivalent to the interest rate the business would have had to pay if the business had purchased the asset instead. The residual value, if any, is included in the amount of this debt, which is known as a capitalized lease liability.2
For example, assume a company signed a two-year lease with quarterly payments of $1,000 and no residual value. Alternatively, it could have purchased the asset and financed it through a bank loan for 8% quarterly. Therefore, applying Formula 11.5, the present value of the eight $1,000 payments discounted at 8% quarterly is $7,471.99. This is the amount that appears on the balance sheet.
The Formula
A lease is an annuity due involving some special characteristics. To solve a leasing situation you can use any of the Chapter 11 annuity due formulas.
How It Works
The typical unknown variable in a leasing situation is the present value, future value, lease payment amount, or lease interest rate. To solve a lease for those variables you follow the exact same steps as found in Section 11.2, Section 11.3, Section 11.4, and Section 11.6.
Things To Watch Out For
Here are some of the most common mistakes made when working with leases:
- Cash Flow Violation. This mistake occurs when the cash flow sign convention on the calculator or in Excel is violated. From the perspective of the lessee, the present value of the lease is a positive number since the lessee is receiving the asset. Payments on the lease are therefore negative. The future value or residual value of the lease (if other than zero) is also a negative number, since it represents the amount still owing on the asset if the lessee wishes to purchase it.
- Confusing the Present Value and the Purchase Price. When calculating the present value of the lease, be careful to answer the question that is asked. If you need to know how much money was financed for the lease, this is your present value, whereas if you need to know the purchase price of the asset, you must add any down payment to the present value to arrive at the right answer.
Assume two cars are financed at the same amount at the same interest rate for the same period of time, but one is leased and the other is purchased. Are the lease payments larger than, smaller than, or equal to the purchase payments?
- Answer
-
Smaller. The lease has a residual value at the end, meaning the lease payments do not have to pay as much principal as would be the case under the purchase payments.
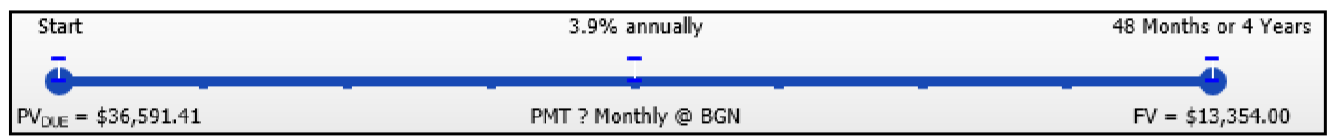
Mazda is advertising a new Mazda3 Sport with an MSRP inclusive of all taxes, deliveries, and fees for $39,091.41. It offers a 48-month lease with a lease rate of 3.9% effectively. The residual value of the vehicle is $13,354.00. Determine the monthly lease payments if a $2,500 down payment is made.
Solution
Step 1:
This is a leasing question, so payments are made at the beginning of each period. Monthly payments with annual compounding create a general annuity due. Calculate the annuity payment amount (\(PMT\)).
What You Already Know
Step 1 (continued):
The timeline for the vehicle lease appears below.
Step 2:
\(PV_{DUE}\) =$39,091.41−$2,500.00 = $36,591.41, \(IY\) = 3.9%, \(CY\) = 1, \(PY\) = 12, Years = 4, \(FV\) = $13,354.00
How You Will Get There
Step 3:
Apply Formula 9.1.
Step 4:
The future value needs to be moved to the start, the same date as \(PV_{DUE}\). Apply Formulas 9.2 and 9.3 (rearranged for \(PV\)). The calculated \(PV\) is money the lease is not required to pay off, so it is subtracted from \(PV_{DUE}\) to arrive at the amount required by the annuity.
Step 5:
Apply Formulas 11.1 and 11.5 (rearranged for \(PMT\)) using the calculated step 4 \(PV_{DUE}\) amount.
Perform
Step 3:
\(i=3.9 \% / 1=3.9 \%\)
Step 4:
\(N=1 \times 4=4 \text { compounds, } \$ 13,354=PV(1+0.039)^{4}\)
\(\text { new } PV_{DUE}=\$ 36,591.41-\$ 11,459.06497=\$ 25,132.34503 \)
Step 5:
\(N=12 \times 4=48 \text { payments }\)
\[\$ 25,132.34503=PMT\left[\dfrac{1-\left[\dfrac{1}{(1+0.039)^{\frac{1}{12}}}\right]^{48}}{(1+0.039)^{\frac{1}{12}}-1}\right] \times(1+0.039)^{\frac{1}{12}} \nonumber \]
\[\$ 25,132.34503=PMT\left[\dfrac{0.141900}{0.003193}\right] \times 1.003193 \nonumber \]
\[PMT=\dfrac{\$ 25,132.34503}{44.578554}=\$ 563.78 \nonumber \]
Calculator Instructions
| Mode | N | I/Y | PV | PMT | FV | P/Y | C/Y |
|---|---|---|---|---|---|---|---|
| BGN | 48 | 3.9 | 36591.41 | Answer: -563.776579 | -13354 | 12 | 1 |
With $2,500 down on this vehicle, a four-year lease has beginning-of-month payments of $563.78.
Bradford Enterprises just updated its computer mainframe systems and leased its new equipment under a two-year contract requiring fixed quarterly payments of $17,300. Alternatively, it could have borrowed the money through a financial institution at 6.5% compounded semi-annually. What liability amount (the capitalized lease liability) should be recorded on the company's balance sheet
- on the day the contract was signed?
- after five payments have been made?
Solution
Step 1:
This is a leasing question, so payments are made at the beginning of the period. Quarterly payments with semi-annual compounding create a general annuity due. Calculate the present value (\(PV_{DUE}\)) at two time points— today and after five payments.
What You Already Know
Step 1 (continued):
The timeline for the computer equipment lease appears below.
Step 2:
\(FV\) = $0, \(IY\) = 6.5%, \(CY\) =2, \(PMT\) = $17,300, \(PY\) = 4, Years = 2
How You Will Get There
Step 3:
Apply Formula 9.1.
Step 4:
Since \(FV\) = $0, skip this step.
Step 5:
Apply Formulas 11.1 and 11.5 twice (for both today and after five payments).

Perform
Step 3:
\(i=6.5 \% / 2=3.25 \% \)
Step 5:
- For today, \(N=4 \times 2=8\) payments \[PV_{DUE}=\$ 17,300\left[\dfrac{1-\left[\dfrac{1}{(1+0.0325)^{\frac{2}{4}}}\right]^{8}}{(1+0.0325)^{\frac{2}{4}}-1}\right] \times(1+0.0325)^{\frac{2}{4}}=\$ 130,954.37 \nonumber \]
- After five payments, \(N=4 \times 3 / 4=3\) payments left \[PV_{DUE}=\$ 17,300\left[\dfrac{1-\left[\dfrac{1}{(1+0.0325)^{\frac{2}{4}}}\right]^{3}}{(1+0.0325)^{\frac{2}{4}}-1}\right] \times(1+0.0325)^{\frac{2}{4}}=\$ 51,080.99 \nonumber \]
Calculator Instructions
| Calculation | Mode | N | I/Y | PV | PMT | FV | P/Y | C/Y |
|---|---|---|---|---|---|---|---|---|
| Today | BGN | 8 | 6.5 | Answer: 130,954.37 | -17300 | 0 | 4 | 2 |
| After 5 payments | \(\surd\) | 3 | \(\surd\) | Answer: 51,080.99 | \(\surd\) | \(\surd\) | \(\surd\) | \(\surd\) |
On the day that the computer equipment is leased, the accountants will record a capital lease liability of $130,954.37. This amount will reduce with each payment, such that after five payments the liability would be $51,080.99.
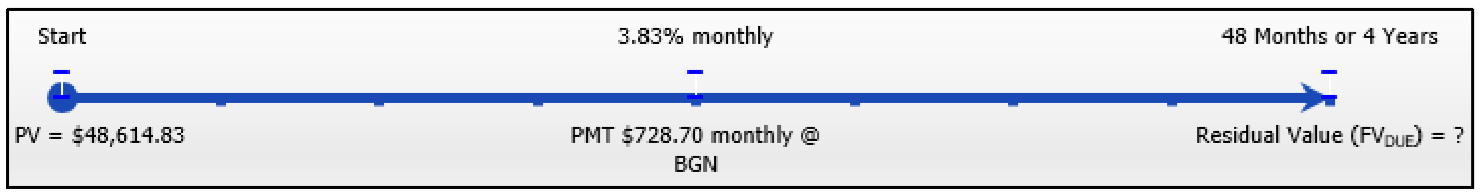
A Nissan 370Z Roadster A7 advertised in the local paper offers monthly payments of $728.70 for 48 months at 3.83% compounded monthly. The lease requires a $5,000 down payment, and the vehicle has an MSRP (including all fees and taxes) of $53,614.83. What residual value is used in the calculation?
Solution
Step 1:
This is a leasing question, so payments are made at the beginning of each period. Monthly payments with monthly compounding create a simple annuity due. Calculate its residual value, which is the same thing as the future value (\(FV_{DUE}\)).
What You Already Know
Step 1 (continued):
The timeline for the vehicle lease appears below.
Step 2:
\(PV\) = $53,614.83 − $5,000 = $48,614.83, \(IY\) = 3.83%, \(CY\) =12, \(PMT\) = $728.70, \(PY\) = 12, Years = 4
How You Will Get There
Step 3:
Apply Formula 9.1.
Step 4:
For the \(PV\), apply Formulas 9.2 and 9.3.
Step 5:
Apply Formulas 11.1 and 11.2. The residual value is the difference between the answers to step 4 (what the lessee owes) and step 5 (what the lessee paid).
Perform
Step 3:
\(i=3.83 \% / 12=0.3191 \overline{6} \%\)
Step 4:
\(N=12 \times 4=48 \text { compounds}; FV=\$ 48,614.83(1+0.003191)^{48}=\$ 56,649.58522 \)
Step 5:
\(N=12 \times 4=48 \text { payments }\)
\[\begin{aligned}FV_{DUE}=\$ 728.70 &\left[\dfrac{\left[(1+0.003191)^{\frac{12}{12}}\right]^{48}-1}{(1+0.003191)^{\frac{12}{12}}-1}\right] \times(1+0.003191)^{\frac{12}{12}} \\ &=\$ 37,854.63271 \end{aligned} \nonumber \]
\[\text { Residual Value }=\$ 56,649.58522-\$ 37,854.63721=\$ 18,794.95 \nonumber \]
Calculator Instructions
| Mode | N | I/Y | PV | PMT | FV | P/Y | C/Y |
|---|---|---|---|---|---|---|---|
| BGN | 48 | 3.83 | 48614.83 | -728.7 | Answer: -18,794.95252 | 12 | 12 |
In determining the lease payments, Nissan expects its Roadster to have a residual value of $18,794.95 after the four year lease term expires. The owner will either have to pay this amount or give the keys back.
An advertisement during The Bachelorette tells you that you can lease a Dodge Challenger SE for $161.00 biweekly for five years. The MSRP of the vehicle is $26,480, and a $1,500 down payment is required. If the residual value of the vehicle is $8,146.16, what annually compounded interest rate does the calculation use?
Solution
Step 1:
This is a leasing question, so payments are made at the beginning of each period. Monthly payments with monthly compounding create a simple annuity due. Calculate the annually compounded interest rate (\(IY\)) with \(CY\) = 1.
What You Already Know
Step 1 (continued):
The timeline for the vehicle lease appears below.

Step 2:
\(PV_{DUE}\) = $26,480 − $1,500 = $24,980, \(CY\) = 1, \(PMT\) = $161, \(PY\) = 26, Years = 5, \(FV\) = $8,146.16
How You Will Get There
Step 3:
Apply Formulas 11.1 and 11.5. Enter this information into the calculator to solve for \(IY\) (which automatically applies Formula 9.1 to convert the \(i\) to an \(IY\)).
Perform
Step 3:
\(N=26 \times 5=130 \text { payments }\)
\[\$ 24,980=\$ 161\left[\dfrac{1-\left[\dfrac{1}{(1+i)^{\frac{1}{26}}}\right]^{130}}{(1+i)^{\frac{1}{26}}-1}\right] \times(1+i)^{\frac{1}{26}} \nonumber \]
See calculator instructions for the solution. \(IY\) = 4.99%
Calculator Instructions
| Mode | N | I/Y | PV | PMT | FV | P/Y | C/Y |
|---|---|---|---|---|---|---|---|
| BGN | 130 | Answer: 4.989999 | 24980 | -161 | -8146.16 | 26 | 1 |
To arrive at payments of $161 biweekly on a Dodge Challenger with $1,500 down and a residual value of $8,146.16, Dodge is using a 4.99% compounded annually interest rate.
References
- Statistics Canada, New Motor Vehicle Sales, June 2011 (Catalogue no. 63-007-X, p. 15), publications.gc.ca/collection...llection_2011/statcan/63-007-X/63-007-x2011006-eng.pdf (accessed August 18, 2013).
- For illustrative purposes this textbook makes some simplifying assumptions:
- Accounting rules require the asset to be recorded at the present value of the minimum lease obligations or its fair market value, whichever is less. The examples in this book assume that the present value is the lesser number.
- Accounting rules require at least one of four specific conditions to be met for a lease to qualify as a capital lease. The examples assume that this criterion is met.
- Issues involving capital leases such as depreciation or executory costs are ignored.


