Ch 1.2 part 1 Types of Data, Summarize Categorical data, Percent Review
- Page ID
- 15874
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Chapter 1.2 Data, sampling and Variation
Types of Variable:
Categorical: name, label or a result of categorizing attributes. Also known as qualitative variable.
Quantitative: counts or numerical measurement with units.
Types of Quantitative data:
Discrete: counts or numbers that takes on finite values.
Continuous: measurement data that can have infinitely many possible values between two values not limited by measurement device.
Level of measurement:
· Nominal scale level: categorical data with no natural ordering
· Ordinal scale level: categorical data with a natural ordering, difference and mean are not meaningful.
· Interval scale level: quantitative data with no natural zero. Zero value is a mark only. Difference is meaningful but ratio is not meaningful.
· Ratio scale level: quantitative data with natural zero. Difference and ratio is meaningful.
Note: Nominal and Ordinal data are usually summarized by proportion of the categories. There is no meaning to the mean.
Ex1 classify the data as quantitative or categorical, discrete or continuous if it is quantitative. Classify by level of measurement also.
a) The number of students in a zoom meeting. ____ quantitative discrete, ratio
b) The major of study of a student._____ categorical, nominal
c) The student id of a student._____categorical, ordinal
d) Jersey number of a basketball player.______ categorical, ordinal
e) The duration (in minutes) it takes to finish a homework.______quantitative continuous, ratio
f) Grade of an exam._____categorical, ordinal
g) Daily high temperature of a city. _____quantitative continuous, interval.
Percent and Decimal:
Decimal → Percentage:
Move decimal point to the right by 2 places.
Example: 0.75 = 75% 0.0178 = 1.78%
Percentage → decimal.
move decimal point to the left by 2 places.
Example: 25% = 0.25, 4% = 0.04 0.1% = 0.001
Find Percent:
\( \dfrac{part}{whole} \) → decimal → percent
Find Percent of an amount: To find percent of an amount, replace the % with decimal notation, interpret “of” to be multiplication.
Example:
6% of 1200 = 0.06 × 1200 = 72
12% of 2418 = 0.12× 2418 =290.16
Exact value of 12% of 2418 adults = 290.16 (no rounding)
Actual value of 12% of 2418 adults = 290 adults
Summarizing Categorical data.
Proportions (relative frequencies) are used to summarize Categorical data. A proportion will be calculated for each category and presented as a table
Ex 1.


Graphs for categorical data.
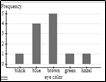 1) Bar Graph: Use bars of equal width to show frequencies of categories. May or may not be separated by spaces.
1) Bar Graph: Use bars of equal width to show frequencies of categories. May or may not be separated by spaces.
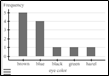 2) Pareto graph: A Bar graph where bars are arranged in descending order of frequencies, so it is easier to compare difference categories
2) Pareto graph: A Bar graph where bars are arranged in descending order of frequencies, so it is easier to compare difference categories
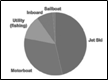 3) Pie Chart – show categorical data as slices of a circle. each slice is proportional to the frequency count for the category. Show composition of the whole.
3) Pie Chart – show categorical data as slices of a circle. each slice is proportional to the frequency count for the category. Show composition of the whole.
How to draw a bar graph, pie chart from frequency table:
Use Excel, select the category and frequency or relative frequency column, insert chart.
Note: When Categories proportion does not add up to 100%, a bar graph is appropriate, but a pie chart is not.
A total percentage more than 100% indicates subjects are in more than one category.
A total percentage less than 100% indicates a missing category such as the “other “category.
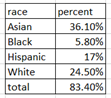
Ex1: Is it appropriate the graph the following table by bar graph, pie chart?
 The total percent for the first frequency table is more than 100%, it is collecting percent of more than one type of variable.
The total percent for the first frequency table is more than 100%, it is collecting percent of more than one type of variable.
It should not be graphed in bar graph or pie chart.
The total percent is less than 100%, a pie chart is not appropriate.