17.1: Chi Square Distribution

Last updated
Save as PDF

Page ID: 2188

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Learning Objectives

Define the Chi Square distribution in terms of squared normal deviates
Describe how the shape of the Chi Square distribution changes as its degrees of freedom increase

A standard normal deviate is a random sample from the standard normal distribution. The Chi Square distribution is the distribution of the sum of squared standard normal deviates. The degrees of freedom of the distribution is equal to the number of standard normal deviates being summed. Therefore, Chi Square with one degree of freedom, written as \(\chi ^2(1)\), is simply the distribution of a single normal deviate squared. The area of a Chi Square distribution below \(4\) is the same as the area of a standard normal distribution below \(2\), since \(4\) is \(2^2\).

Consider the following example.

Example \(\PageIndex{1}\)

You sample two scores from a standard normal distribution, square each score, and sum the squares. What is the probability that the sum of these two squares will be six or higher?

Solution

Since two scores are sampled, the answer can be found using the Chi Square distribution with two degrees of freedom. A Chi Square calculator can be used to find that the probability of a Chi Square (with \(2 df\)) being six or higher is \(0.050\).

The mean of a Chi Square distribution is its degrees of freedom. Chi Square distributions are positively skewed, with the degree of skew decreasing with increasing degrees of freedom. As the degrees of freedom increases, the Chi Square distribution approaches a normal distribution. Figure \(\PageIndex{1}\) shows density functions for three Chi Square distributions. Notice how the skew decreases as the degrees of freedom increases.

Figure \(\PageIndex{1}\): Chi Square distributions with \(2\), \(4\), and \(6\) degrees of freedom

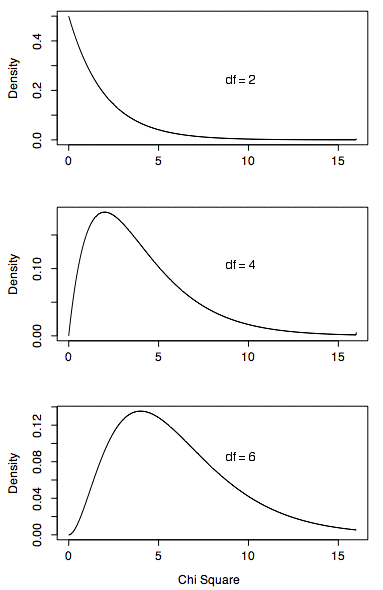
The Chi Square distribution is very important because many test statistics are approximately distributed as Chi Square. Two of the more common tests using the Chi Square distribution are tests of deviations of differences between theoretically expected and observed frequencies (one-way tables) and the relationship between categorical variables (contingency tables). Numerous other tests beyond the scope of this work are based on the Chi Square distribution.